Стерадијан
Стерадијан (ste од грчког stereos, просторан; симбол: sr) је СИ изведена јединица за просторни угао и тродимензионални еквивалент радијана. Стерадијан се дефинише као "просторни угао код центра кугле полупречника r затворен делом површине кугле са површином r2." Пошто је површина ове сфере 4πr2, онда дефиниција имплицира да кугла има 4π стерадијана. Стерадијан се такође може назвати квадрираним радијаном.[1][2][3]
| Стерадијан | |
|---|---|
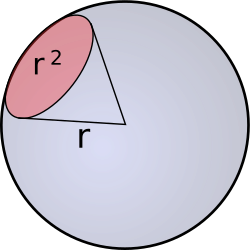
 Графички приказ једног стерадијана. Сфера има полупречник r, и у овом случају површина A означене сферне капе је r2. Пуни угао Ω једнак је [A/r2] sr што је 1 sr у овом примеру. Цела сфера има просторни угао од 4π sr. | |
| Информације о јединици | |
| Систем | СИ изведена јединица |
| Јединица | Просторни угао |
| Симбол | sr |
| Јединична претварања | |
| 1 sr у ... | ... је једнак са ... |
| СИ основне јединице | 1 m²/m² |
Стерадијан је такође једнак сферној површини полигона који има додатак угла од једног радијана, до 1/4π целе кугле, или до (180/π)2 односно 3282,80635 квадратних степени. Стерадијан је првобитно био СИ допунска јединица, али је ова категорија укинута из СИ система 1995. године.[4]
Стерадијан, као и радијан,[5] је бездимензионална јединица,[6][7][8] количник напрамне површине и квадрата њене удаљености од центра. Бројилац и именилац овог односа имају квадратну дужину димензије (тј. L2/L2 = 1, без димензија). Корисно је, међутим, разликовати бездимензионалне величине различите природе, те се симбол „sr“ користи за означавање просторног угла. На пример, интензитет зрачења се може мерити у ватима по стерадијану (W⋅sr−1). Стерадијан је раније био допунска јединица СИ, али је ова категорија укинута 1995. и стерадијан се сада сматра јединицом изведеном из СИ.[9]

Дефиниција
уредиСтерадијан се може дефинисати као просторни угао који је у центру јединичне сфере састављен јединичном сфером на њеној површини. За општу сферу полупречника r, било који део њене површине са површином A = r2 обухвата један стерадијан у њеном центру.[10]
Просторни угао је повезан са површином коју сече из сфере:
где
- Ω је просторни угао
- A је површина сферне капе, ,
- r је полупречник сфере, и
- sr је јединица, стерадијан.
Пошто је површина A сфере 4πr2, дефиниција имплицира да сфера тежи 4π стерадијана (≈ 12,56637 sr) у свом центру, или да стерадијан тежи 1/4π (≈ 0,07958) сфере. По истом аргументу, максимални просторни угао који се може подвести у било којој тачки је 4π sr.
Друга својства
уредиАко је A = r2, то одговара површини сферне капе (A = 2πrh) (где h представља „висину“ капе) и важи однос h/r = 1/2π. Према томе, у овом случају, један стерадијан одговара равном (тј. радијанском) углу попречног пресека једноставног конуса који пружа раван угао 2θ, при чему је θ дат као:
Овај угао одговара углу равног отвора бленде од 2θ ≈ 1,144 rad или 65,54°.
Стерадијан је такође једнак сферној површини полигона чији је угаони вишак 1 радијан, до 1/4π целе сфере или до (180°/π)2
≈ 3282,80635 квадратних степени.
Пуни угао конуса чији попречни пресек пружа угао 2θ је:
- .
СИ умношци
уредиМилистерадијани (msr) и микростерадијани (μsr) се повремено користе за описивање снопова светлости и честица.[11][12] Други умношци се ретко користе.
Референце
уреди- ^ Stutzman, Warren L; Thiele, Gary A (2012-05-22). Antenna Theory and Design. ISBN 978-0-470-57664-9.
- ^ Woolard, Edgar (2012-12-02). Spherical Astronomy. ISBN 978-0-323-14912-9.
- ^ International Bureau of Weights and Measures (2006), The International System of Units (SI) (PDF) (8th изд.), ISBN 92-822-2213-6
- ^ Suplee, Curt (2. 7. 2009). „Special Publication 811”.
- ^ „Resolution 8 of the CGPM at its 20th Meeting (1995)”. Bureau International des Poids et Mesures. Архивирано из оригинала 2018-12-25. г. Приступљено 2014-09-23.
- ^ „1.8 (1.6) quantity of dimension one dimensionless quantity”. International vocabulary of metrology — Basic and general concepts and associated terms (VIM). ISO. 2008. Приступљено 2011-03-22.
- ^ „SI Brochure: The International System of Units, 9th Edition”. International Bureau of Weights and Measures (BIPM). ISBN 978-92-822-2272-0.
- ^ Mohr, Peter J.; Phillips, William D. (2015-06-01). „Dimensionless units in the SI”. Metrologia (на језику: енглески). 52.
- ^ „Resolution 8 of the CGPM at its 20th Meeting (1995)”. Bureau International des Poids et Mesures. Приступљено 2014-09-23.
- ^ "Steradian", McGraw-Hill Dictionary of Scientific and Technical Terms, fifth edition, Sybil P. Parker, editor in chief. McGraw-Hill, (1997) ISBN 0-07-052433-5.
- ^ Stephen M. Shafroth, James Christopher Austin, (1997). Accelerator-based Atomic Physics: Techniques and Applications. стр. 333. ISBN 1563964848.
- ^ R. Bracewell, Govind Swarup, "The Stanford microwave spectroheliograph antenna, a microsteradian pencil beam interferometer" IRE Transactions on Antennas and Propagation 9:1:22-30 (1961)
Литература
уреди- E Richard Cohen; Tom Cvitas; Jeremy G Frey; Bertil Holstrom; John W Jost, ур. (2007). Quantities, Units and Symbols in Physical Chemistry (PDF). International Union of Pure and Applied Chemistry (3. изд.). Royal Society of Chemistry; 3rd edition. ISBN 0854044337.
- International Union of Pure and Applied Chemistry (1993). Quantities, Units and Symbols in Physical Chemistry, 2nd edition, Oxford: Blackwell Science. ISBN 0-632-03583-8. Electronic version.
- I. Mills; Tomislav Cvitas, Klaus Homann, Nikola Kallay, IUPAC (јун 1993). Quantities, Units and Symbols in Physical Chemistry (2nd изд.). Blackwell Science Inc. стр. 72.
- Hall, Arthur Graham; Frink, Fred Goodrich (јануар 1909). „Chapter VII. The General Angle [55] Signs and Limitations in Value. Exercise XV.”. Написано на Ann Arbor, Michigan, USA. Trigonometry. Part I: Plane Trigonometry. New York, USA: Henry Holt and Company / Norwood Press / J. S. Cushing Co. - Berwick & Smith Co., Norwood, Massachusetts, USA. стр. 73. Приступљено 2017-08-12.
- International Bureau of Weights and Measures (20. 5. 2019), The International System of Units (SI) (PDF) (9th изд.), ISBN 978-92-822-2272-0, Архивирано (PDF) из оригинала 8. 5. 2021. г.
- Brinsmade, J. B. (децембар 1936). „Plane and Solid Angles. Their Pedagogic Value When Introduced Explicitly”. American Journal of Physics. 4 (4): 175—179. Bibcode:1936AmJPh...4..175B. doi:10.1119/1.1999110.
- Romain, Jacques E. (јул 1962). „Angle as a fourth fundamental quantity”. Journal of Research of the National Bureau of Standards, Section B. 66B (3): 97. doi:10.6028/jres.066B.012 .
- Eder, W E (јануар 1982). „A Viewpoint on the Quantity "Plane Angle"”. Metrologia. 18 (1): 1—12. Bibcode:1982Metro..18....1E. doi:10.1088/0026-1394/18/1/002.
- Torrens, A B (1. 1. 1986). „On Angles and Angular Quantities”. Metrologia. 22 (1): 1—7. Bibcode:1986Metro..22....1T. doi:10.1088/0026-1394/22/1/002.
- Brownstein, K. R. (јул 1997). „Angles—Let's treat them squarely”. American Journal of Physics. 65 (7): 605—614. Bibcode:1997AmJPh..65..605B. doi:10.1119/1.18616.
- Lévy-Leblond, Jean-Marc (септембар 1998). „Dimensional angles and universal constants”. American Journal of Physics. 66 (9): 814—815. Bibcode:1998AmJPh..66..814L. doi:10.1119/1.18964.
- Foster, Marcus P (1. 12. 2010). „The next 50 years of the SI: a review of the opportunities for the e-Science age”. Metrologia. 47 (6): R41—R51. doi:10.1088/0026-1394/47/6/R01.
- Mohr, Peter J; Phillips, William D (1. 2. 2015). „Dimensionless units in the SI”. Metrologia. 52 (1): 40—47. Bibcode:2015Metro..52...40M. arXiv:1409.2794 . doi:10.1088/0026-1394/52/1/40 .
- Quincey, Paul (1. 4. 2016). „The range of options for handling plane angle and solid angle within a system of units”. Metrologia. 53 (2): 840—845. Bibcode:2016Metro..53..840Q. doi:10.1088/0026-1394/53/2/840.
- Mills, Ian (1. 6. 2016). „On the units radian and cycle for the quantity plane angle”. Metrologia. 53 (3): 991—997. Bibcode:2016Metro..53..991M. doi:10.1088/0026-1394/53/3/991.
- Quincey, Paul (1. 10. 2021). „Angles in the SI: a detailed proposal for solving the problem”. Metrologia. 58 (5): 053002. Bibcode:2021Metro..58e3002Q. S2CID 236547235. arXiv:2108.05704 . doi:10.1088/1681-7575/ac023f.
- Leonard, B P (1. 10. 2021). „Proposal for the dimensionally consistent treatment of angle and solid angle by the International System of Units (SI)”. Metrologia. 58 (5): 052001. Bibcode:2021Metro..58e2001L. S2CID 234036217. doi:10.1088/1681-7575/abe0fc.
- Mohr, Peter J; Shirley, Eric L; Phillips, William D; Trott, Michael (23. 6. 2022). „On the dimension of angles and their units”. Metrologia. arXiv:2203.12392 . doi:10.1088/1681-7575/ac7bc2 .
- Buckingham, E. (1914). „On physically similar systems; illustrations of the use of dimensional equations”. Physical Review. 4 (4): 345—376. Bibcode:1914PhRv....4..345B. doi:10.1103/PhysRev.4.345. hdl:10338.dmlcz/101743 .
- „SI units need reform to avoid confusion”. Nature (на језику: енглески). 548 (7666): 135. август 2017. Bibcode:2017Natur.548R.135.. ISSN 1476-4687. PMID 28796224. S2CID 4444368. doi:10.1038/548135b.
- Wendl, Michael C. (септембар 2017). „Don't tamper with SI-unit consistency”. Nature (на језику: енглески). 549 (7671): 160. ISSN 1476-4687. PMID 28905893. S2CID 52806576. doi:10.1038/549160d.
- „BIPM Consultative Committee for Units (CCU), 15th Meeting” (PDF). 17—18. 4. 2003. Архивирано из оригинала (PDF) 2006-11-30. г. Приступљено 2010-01-22.
- „BIPM Consultative Committee for Units (CCU), 16th Meeting” (PDF). Архивирано из оригинала (PDF) 2006-11-30. г. Приступљено 2010-01-22.
- Dybkaer, René (2004). „An ontology on property for physical, chemical, and biological systems”. APMIS Suppl. (117): 1—210. PMID 15588029.
- Rothstein, S. (2017). Semantics for Counting and Measuring. Key Topics in Semantics and Pragmatics. Cambridge University Press. стр. 206. ISBN 978-1-107-00127-5. Приступљено 2021-11-30.
- Berch, D.B.; Geary, D.C.; Koepke, K.M. (2015). Development of Mathematical Cognition: Neural Substrates and Genetic Influences. ISSN. Elsevier Science. стр. 13. ISBN 978-0-12-801909-2. Приступљено 2021-11-30.
- Protter, Murray H.; Morrey, Charles B., Jr. (1970), College Calculus with Analytic Geometry (2nd изд.), Reading: Addison-Wesley, стр. APP-4, LCCN 76087042
- Lustig, Rolf (1986). „Geometry of four hard fused spheres in an arbitrary spatial configuration”. Molecular Physics. 59 (2): 195—207. Bibcode:1986MolPh..59..195L. doi:10.1080/00268978600102011.
- Gibson, K. D.; Scheraga, Harold A. (1987). „Volume of the intersection of three spheres of unequal size: a simplified formula”. The Journal of Physical Chemistry. 91 (15): 4121—4122. doi:10.1021/j100299a035.
- Gibson, K. D.; Scheraga, Harold A. (1987). „Exact calculation of the volume and surface area of fused hard-sphere molecules with unequal atomic radii”. Molecular Physics. 62 (5): 1247—1265. Bibcode:1987MolPh..62.1247G. doi:10.1080/00268978700102951.
- Petitjean, Michel (1994). „On the analytical calculation of van der Waals surfaces and volumes: some numerical aspects”. Journal of Computational Chemistry. 15 (5): 507—523. doi:10.1002/jcc.540150504.
- Grant, J. A.; Pickup, B. T. (1995). „A Gaussian description of molecular shape”. The Journal of Physical Chemistry. 99 (11): 3503—3510. doi:10.1021/j100011a016.
- Busa, Jan; Dzurina, Jozef; Hayryan, Edik; Hayryan, Shura (2005). „ARVO: A fortran package for computing the solvent accessible surface area and the excluded volume of overlapping spheres via analytic equations”. Computer Physics Communications. 165 (1): 59—96. Bibcode:2005CoPhC.165...59B. doi:10.1016/j.cpc.2004.08.002.
Спољашње везе
уреди- Weisstein, Eric W. „Radian”. mathworld.wolfram.com (на језику: енглески). Приступљено 2020-08-31.