Hi-kvadratna raspodela
U teoriji verovatnoće i statistici, hi-kvadratna raspodela (takođe hi-kvadrat ili χ2-raspodela) sa k stepena slobode je distribucija sume kvadrata k nezavisnih standardno normalnih randomnih promenljivih. Hi-kvadratna distribucija je specijalni slučaj gama distribucije i jedna je od od najšire korištenih distribucija verovatnoće u inferencijskoj statistici, naročito u testiranju hipoteza ili u konstrukciji intervala pouzdanosti.[2][3][4][5] Kada se pravi razlika od opštije necentralne hi-kvadratne raspodele, ova distribucija se ponekad naziva centralnom hi-kvadratnom raspodelom.
Funkcija gustine verovatnoće  | |
Funkcija kumulativne raspodele 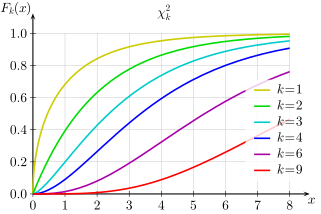 | |
| Notacija | ili |
|---|---|
| Parametri | (poznati kao „stepeni sloboda”) |
| Nositelj | ako je , inače |
| CDF | |
| Prosek | |
| Medijana | |
| Modus | |
| Varijansa | |
| Koef. asimetrije | |
| Kurtoza | |
| Entropija | |
| MGF | |
| CF | [1] |
| PGF | |
Hi-kvadratna raspodela se koristi u uobičajenim hi-kvadratnim testovima[6][7] za adekvatnost uklapanja posmatrane distribucije u teorijski očekivanu, nezavisnost dva kriterijuma klasifikacije kvalitativnih podataka, i procenu intervala pouzdanosti za populaciju standardnih devijacija normalne distribucije iz standardne devijacije uzorka. Mnogi drugi statistički testovi takođe koriste ovu distribuciju, kao što je Fridmanova analiza varijanse po rangovima.
Definicija
уредиAko su Z1, ..., Zk nezavisnе, standardno normalne randomne promenljive, onda je suma njihovih kvadrata,
distribuirana u skladu sa hi-kvadratnom distribucijom sa k stepeni slobode. Ovo se obično označava sa
Hi-kvadratna distribucija ima jedan parametar: k, pozitivni integer koji specificira broj stepeni slobode (broj Zi vrednosti).
Tabela χ2 vrednosti vs p-vrednosti
уредиp-vrednost je verovatnoća opservacije statističkog testa bar kao ekstrema u hi-kvadratnoj distribuciji. Shodno tome, pošto kumulativna funkcija raspodele (CDF) za odgovarajuće stepene slobode (df) daje verovatnoću da je dobijena vrednost manje ekstremna od ove tačke, oduzimanje CDF vrednosti od 1 daje p-vrednost. Mala p-vrednost, ispod izabranog nivoa značaja, ukazuje na statistički značaj, tj. dovoljan dokaz da se odbaci nulta hipoteza. Nivo značaja od 0,05 se često koristi kao granica između značajnih i neznačajnih rezultata.
Donja tabela daje broj p-vrednosti koje odgovaraju sa χ2 za prvih 10 stepeni slobode.
| Stepeni slobode (df) | χ2 vrednost[8] | ||||||||||
|---|---|---|---|---|---|---|---|---|---|---|---|
| 1 | 0,004 | 0,02 | 0,06 | 0,15 | 0,46 | 1,07 | 1,64 | 2,71 | 3,84 | 6,63 | 10,83 |
| 2 | 0,10 | 0,21 | 0,45 | 0,71 | 1,39 | 2,41 | 3,22 | 4,61 | 5,99 | 9,21 | 13,82 |
| 3 | 0,35 | 0,58 | 1,01 | 1,42 | 2,37 | 3,66 | 4,64 | 6,25 | 7,81 | 11,34 | 16,27 |
| 4 | 0,71 | 1,06 | 1,65 | 2,20 | 3,36 | 4,88 | 5,99 | 7,78 | 9,49 | 13,28 | 18,47 |
| 5 | 1,14 | 1,61 | 2,34 | 3,00 | 4,35 | 6,06 | 7,29 | 9,24 | 11,07 | 15,09 | 20,52 |
| 6 | 1,63 | 2,20 | 3,07 | 3,83 | 5,35 | 7,23 | 8,56 | 10,64 | 12,59 | 16,81 | 22,46 |
| 7 | 2,17 | 2,83 | 3,82 | 4,67 | 6,35 | 8,38 | 9,80 | 12,02 | 14,07 | 18,48 | 24,32 |
| 8 | 2,73 | 3,49 | 4,59 | 5,53 | 7,34 | 9,52 | 11,03 | 13,36 | 15,51 | 20,09 | 26,12 |
| 9 | 3,32 | 4,17 | 5,38 | 6,39 | 8,34 | 10,66 | 12,24 | 14,68 | 16,92 | 21,67 | 27,88 |
| 10 | 3,94 | 4,87 | 6,18 | 7,27 | 9,34 | 11,78 | 13,44 | 15,99 | 18,31 | 23,21 | 29,59 |
| P vrednost (verovatnoća) | 0,95 | 0,90 | 0,80 | 0,70 | 0,50 | 0,30 | 0,20 | 0,10 | 0,05 | 0,01 | 0,001 |
Ove vrednosti se mogu izračunati procenom funkcije kvantila (takođe poznate kao „inverzni CDF” ili „ICDF”) raspodele hi-kvadrata;[9] e. g., χ2 ICDF za p = 0,05 i df = 7 daje 14,06714 ≈ 14,07 kao u gornjoj tabeli.
Reference
уреди- ^ M.A. Sanders. „Characteristic function of the central chi-square distribution” (PDF). Архивирано из оригинала (PDF) 2011-07-15. г. Приступљено 2009-03-06.
- ^ Abramowitz, Milton; Stegun, Irene Ann, ур. (1983) [јун 1964]. „поглавље 26”. Handbook of Mathematical Functions with Formulas, Graphs, and Mathematical Tables. Applied Mathematics Series. 55 (Ninth reprint with additional corrections of tenth original printing with corrections (December 1972); first изд.). Washington D.C.; New York: United States Department of Commerce, National Bureau of Standards; Dover Publications. стр. 940. ISBN 978-0-486-61272-0. LCCN 64-60036. MR 0167642. LCCN 65-12253.
- ^ NIST (2006). Engineering Statistics Handbook – Chi-Squared Distribution
- ^ Johnson, N. L.; Kotz, S.; Balakrishnan, N. (1994). „Chi-Square Distributions including Chi and Rayleigh”. Continuous Univariate Distributions. 1 (Second изд.). John Wiley and Sons. стр. 415—493. ISBN 978-0-471-58495-7.
- ^ Mood, Alexander; Graybill, Franklin A.; Boes, Duane C. (1974). Introduction to the Theory of Statistics (Third изд.). McGraw-Hill. стр. 241–246. ISBN 978-0-07-042864-5.
- ^ „Chi-Square - Sociology 3112 - Department of Sociology - The University of utah”. soc.utah.edu. Приступљено 2022-11-12.
- ^ Pearson, Karl (1900). „On the criterion that a given system of deviations from the probable in the case of a correlated system of variables is such that it can be reasonably supposed to have arisen from random sampling” (PDF). Philosophical Magazine. Series 5. 50 (302): 157—175. doi:10.1080/14786440009463897.
- ^ Chi-Squared Test Архивирано на сајту Wayback Machine (18. новембар 2013) Table B.2. Dr. Jacqueline S. McLaughlin at The Pennsylvania State University. In turn citing: R. A. Fisher and F. Yates, Statistical Tables for Biological Agricultural and Medical Research, 6th ed., Table IV. Two values have been corrected, 7.82 with 7.81 and 4.60 with 4.61
- ^ R Tutorial: Chi-squared Distribution
Literatura
уреди- Hald, Anders (1998). A history of mathematical statistics from 1750 to 1930. New York: Wiley. ISBN 978-0-471-17912-2.
- Elderton, William Palin (1902). „Tables for Testing the Goodness of Fit of Theory to Observation”. Biometrika. 1 (2): 155—163. doi:10.1093/biomet/1.2.155.
- Hazewinkel Michiel, ур. (2001). „Chi-squared distribution”. Encyclopaedia of Mathematics. Springer. ISBN 978-1556080104.
- Pierre Simon de Laplace (1812). Analytical Theory of Probability.
- A. Kolmogoroff (1933). Grundbegriffe der Wahrscheinlichkeitsrechnung. ISBN 978-3-642-49888-6. doi:10.1007/978-3-642-49888-6.
- Patrick Billingsley (1979). Probability and Measure. New York, Toronto, London: John Wiley and Sons.
- Olav Kallenberg; Foundations of Modern Probability, 2nd ed. Springer Series in Statistics. (2002). 650 pp. ISBN 0-387-95313-2
- Henk Tijms (2004). Understanding Probability. Cambridge Univ. Press.
- Olav Kallenberg; Probabilistic Symmetries and Invariance Principles. Springer -Verlag, New York (2005). 510 pp. ISBN 0-387-25115-4
- Durrett, Rick (2019). Probability: Theory and Examples, 5th edition. UK: Cambridge University Press. ISBN 9781108473682.
- Gut, Allan (2005). Probability: A Graduate Course. Springer-Verlag. ISBN 0-387-22833-0.
- Pearson, Karl (1893). „Contributions to the mathematical theory of evolution [abstract]”. Proceedings of the Royal Society. 54: 329—333. JSTOR 115538. doi:10.1098/rspl.1893.0079 .
- Pearson, Karl (1895). „Contributions to the mathematical theory of evolution, II: Skew variation in homogeneous material”. Philosophical Transactions of the Royal Society. 186: 343—414. Bibcode:1895RSPTA.186..343P. JSTOR 90649. doi:10.1098/rsta.1895.0010 .
- Pearson, Karl (1901). „Mathematical contributions to the theory of evolution, X: Supplement to a memoir on skew variation”. Philosophical Transactions of the Royal Society A. 197 (287–299): 443—459. Bibcode:1901RSPTA.197..443P. JSTOR 90841. doi:10.1098/rsta.1901.0023 .
- Pearson, Karl (1916). „Mathematical contributions to the theory of evolution, XIX: Second supplement to a memoir on skew variation”. Philosophical Transactions of the Royal Society A. 216 (538–548): 429—457. Bibcode:1916RSPTA.216..429P. JSTOR 91092. doi:10.1098/rsta.1916.0009 .
- Corder, G. W.; Foreman, D. I. (2014), Nonparametric Statistics: A Step-by-Step Approach, New York: Wiley, ISBN 978-1118840313
- Greenwood, Cindy; Nikulin, M. S. (1996), A guide to chi-squared testing, New York: Wiley, ISBN 0-471-55779-X
- Nikulin, M. S. (1973), „Chi-squared test for normality”, Proceedings of the International Vilnius Conference on Probability Theory and Mathematical Statistics, 2, стр. 119—122
- Bagdonavicius, V.; Nikulin, M. S. (2011), „Chi-squared goodness-of-fit test for right censored data”, The International Journal of Applied Mathematics and Statistics, стр. 30—50[потребан је пун навод]
- „Chi-squared Statistic”. Practical Cryptography. Архивирано из оригинала 18. 2. 2015. г. Приступљено 18. 2. 2015.
- „Using Chi Squared to Crack Codes”. IB Maths Resources. British International School Phuket. 15. 6. 2014.
- Ryabko, B. Ya.; Stognienko, V. S.; Shokin, Yu. I. (2004). „A new test for randomness and its application to some cryptographic problems” (PDF). Journal of Statistical Planning and Inference. 123 (2): 365—376. doi:10.1016/s0378-3758(03)00149-6. Приступљено 18. 2. 2015.
- Feldman, I.; Rzhetsky, A.; Vitkup, D. (2008). „Network properties of genes harboring inherited disease mutations”. PNAS. 105 (11): 4323—432. Bibcode:2008PNAS..105.4323F. PMC 2393821 . PMID 18326631. doi:10.1073/pnas.0701722105 .
- „chi-square-tests” (PDF). Архивирано из оригинала (PDF) 29. 6. 2018. г. Приступљено 29. 6. 2018.
Spoljašnje veze
уреди- Earliest Uses of Some of the Words of Mathematics: entry on Chi squared has a brief history
- Course notes on Chi-Squared Goodness of Fit Testing from Yale University Stats 101 class.
- Mathematica demonstration showing the chi-squared sampling distribution of various statistics, e. g. Σx², for a normal population
- Lin, Jinn-Tyan (1988). „Approximating the Cumulative Chi-Square Distribution and its Inverse”. Journal of the Royal Statistical Society. Series D (The Statistician). 37 (1): 3—5. JSTOR 2348373. doi:10.2307/2348373. Simple algorithm for approximating cdf and inverse cdf for the chi-squared distribution with a pocket calculator]
- Values of the Chi-squared distribution

















