Датотека:Surface integral illustration.svg
Величина PNG прегледа за ову SVG датотеку је 512 × 348 пиксела. 5 других резолуција: 320 × 218 пиксела | 640 × 435 пиксела | 1.024 × 696 пиксела | 1.280 × 870 пиксела | 2.560 × 1.740 пиксела.
Оригинална датотека (SVG датотека, номинално 512 × 348 пиксела, величина: 20 kB)
Историја датотеке
Кликните на датум/време да бисте видели тадашњу верзију датотеке.
| Датум/време | Минијатура | Димензије | Корисник | Коментар | |
|---|---|---|---|---|---|
| тренутна | 01:36, 12. децембар 2014. |  | 512 × 348 (20 kB) | McMetrox | Reduced file size |
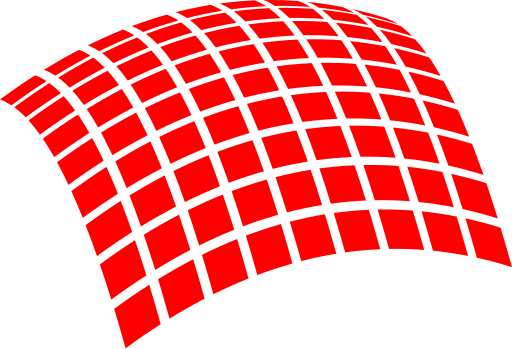
| 00:50, 12. децембар 2014. |  | 512 × 348 (39 kB) | McMetrox | {{Information |Description ={{en|1=The definition of surface integral relies on splitting the surface into small surface elements. Figure 1: The definition of surface integral relies on splitting the surface into small surface elements. Each element... |
Употреба датотеке
2 следеће странице користе ову датотеку:
Глобална употреба датотеке
Други викији који користе ову датотеку:
- Употреба на ar.wiki.x.io
- Употреба на ast.wiki.x.io
- Употреба на bn.wiki.x.io
- Употреба на ca.wiki.x.io
- Употреба на cs.wikibooks.org
- Употреба на cv.wiki.x.io
- Употреба на en.wiki.x.io
- Употреба на eo.wiki.x.io
- Употреба на es.wiki.x.io
- Употреба на fa.wiki.x.io
- Употреба на gl.wiki.x.io
- Употреба на he.wiki.x.io
- Употреба на hu.wiki.x.io
- Употреба на id.wiki.x.io
- Употреба на it.wiki.x.io
- Употреба на ja.wiki.x.io
- Употреба на ka.wiki.x.io
- Употреба на km.wiki.x.io
- Употреба на kn.wiki.x.io
- Употреба на ko.wiki.x.io
- Употреба на nl.wiki.x.io
- Употреба на ro.wiki.x.io
- Употреба на ru.wiki.x.io
- Употреба на simple.wiki.x.io
- Употреба на sq.wiki.x.io
- Употреба на ta.wiki.x.io
- Употреба на te.wiki.x.io
- Употреба на tl.wiki.x.io
- Употреба на tr.wiki.x.io
- Употреба на tt.wiki.x.io
- Употреба на uk.wiki.x.io
- Употреба на vi.wiki.x.io
- Употреба на zh.wiki.x.io